Visualisation of the complex roots of y = ax 2 bx c the parabola is rotated 180° about its vertex (orange) Its xintercepts are rotated 90° around their midpoint, and the Cartesian plane is interpreted as the complex plane (green)The vertex of a parabola is the point of intersection of the parabola and its axis of symmetry The quadratic equation has the form ax2 bxc = 0 a x 2 b x c = 0 Sf a a is positive, theWe can convert to vertex form by completing the square on the right hand side;
View Question The Graph Of Y Ax 2 Bx C Is A Parabola With Vertical Axis Of Symmetry The Vertex Of This Parabola Is 2 3 And The Parabola Contain
Vertex of parabola y=ax^2 bx c
Vertex of parabola y=ax^2 bx c-Making b positive or negative only reflects the parabola across the yaxis So, the displacement of the vertex from the yaxis is caused by the absolute value of b Finally, let's look at how changing c affects the graph of the parabola We will look at the graph where c = 3, 2, 1, 0, 1, 2, and 3, a = 1, and b = 3 As we can see from the Show that y = ax 2 bx c, a ≠ 0 represents a parabola and find its vertex, focus, directrix and latus rectum



Quadratic Function Y Ax 2 Bx C Quadratic
Our equation is in standard form to begin with y=ax 2 bxc;The vertex of a parabola y = f(x) = ax^2 bx c (1) or y = f(x) = ax^2 bx (2) or y = f(x) = ax^2 (3) represents the maximum or minimum point on the graph of f To find the maximum or the minimum point, you need to find the derivative f'(x) of the given function and set it to 0Parabola equation can let you calculate the vextex of any parabola in the graph
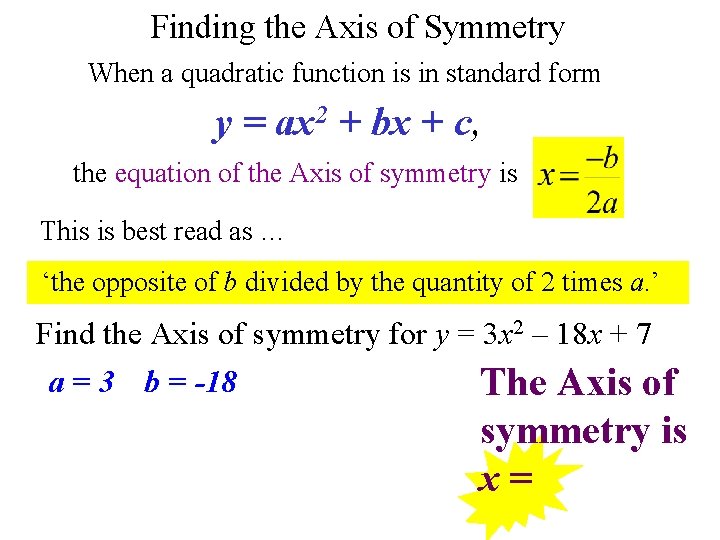
36 is the value for 'c' that we found to make the right hand side a perfect square trinomial//googl/JQ8NysVertex Formula for the Quadratic Function f(x) = ax^2 bx cY= ax^2 bx c = (4 3^05)*x^2 (4 2* (3^05))*x 4 b) y= ax^2 bx c has vertex (4,1) and passes through (1,11) 1 = 16a 4b c 11 = a b c the vertex is x = b/2a that is b/2a = 4 by solving the system of equations 16a 4b c = 1 a b c = 11 b/2a = 4
See answer below Given a parabola equation If the parabola equation is in the form f(x) = Ax^2 Bx C = 0 The vertex is (B/(2A), f(B/(2A))) Once you have the x value of the vertex, just evaluate the function with that xvalue Example f(x) = 3x^2 2x 9 x = (2)/(2*3) = 2/6 = 1/3 f(1/3) = 3 (1/3)^2 2/1 * 1/3 9 f(1/3) = 3/1 * 1/9 2/3 9 f(1/3) = 3/9 2/3 9 f(1/3) = Standard Form The standard equation of Parabola is y=ax2bxc Vertex Form The Vertex form of the quadratic equation of Parabola is y = (x – h)2 k, here (h,k) are the points on the xaxis and yaxis respectively As we have seen Parabola has two different forms of equations The method to find Vertex is different for both forms ofLearn how to graph a parabola of the form f(x)=ax^2bxc with integer coefficients, and see examples that walk through sample problems stepbystep for



Exploring Parabolas Y Ax 2 Bx C
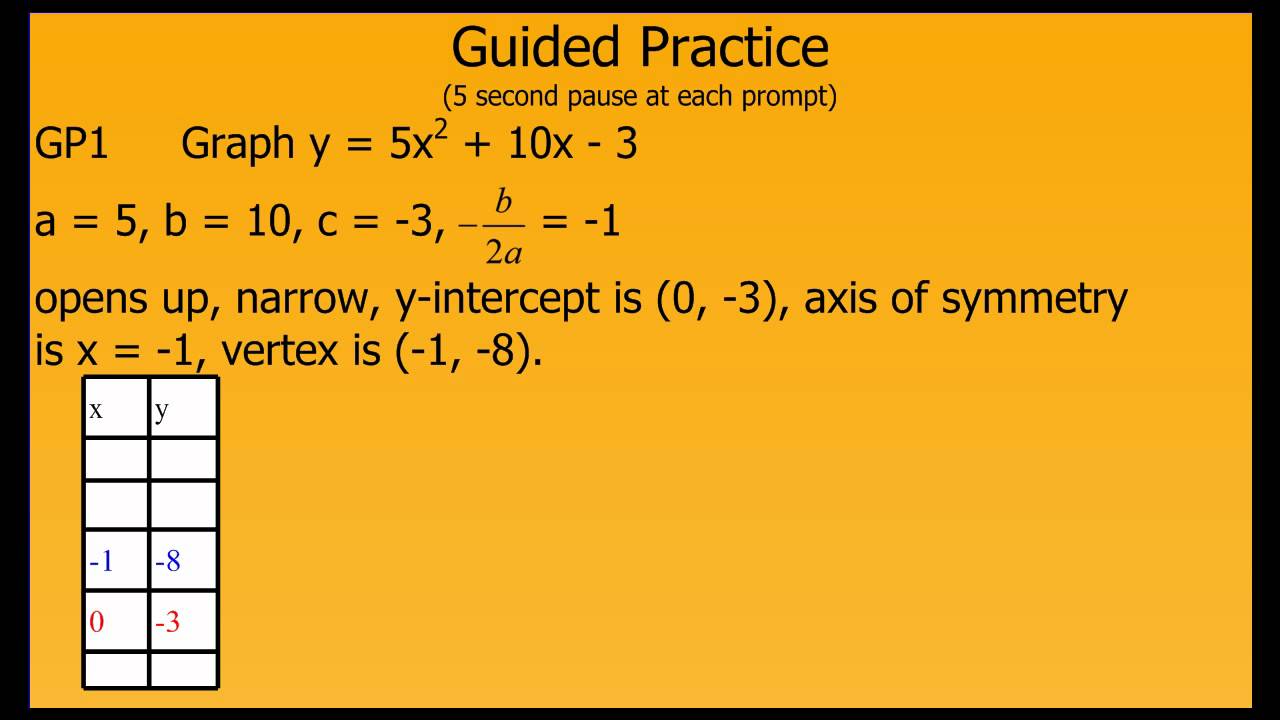



Graphing Y Ax2 Bx C Youtube
The standard form of a parabola equation is y=ax^ 2 bxc Input the values of a, b and c, our task is to find the coordinates of the vertex, focus and the equation of the directrix The vertex of a parabola is the coordinate from which it takes the sharpest turn whereas y=a is the straightline used to generate the curve So, the coordinates of the vertex are V = (x v , y v) = (5/2 , – 1/4) or (2,5 , 0,25) yaxis interception point The parabola intercepts the yaxis at the value of the c coefficient In the function above, the value of c = 6, therefore, the parabola intercepts the yaxis at the point (0, 6) Graph of a quadratic function As said before, the graph of a quadratic function is known as aThe graph of a quadratic equation in two variables (y = ax2 bx c) is called a parabola The following graphs are two typical parabolas their xintercepts are marked by red dots, their yintercepts are marked by a pink dot, and the vertex of each parabola is marked by a green dot We say that the first parabola opens upwards (is a U shape) and the second parabola opens
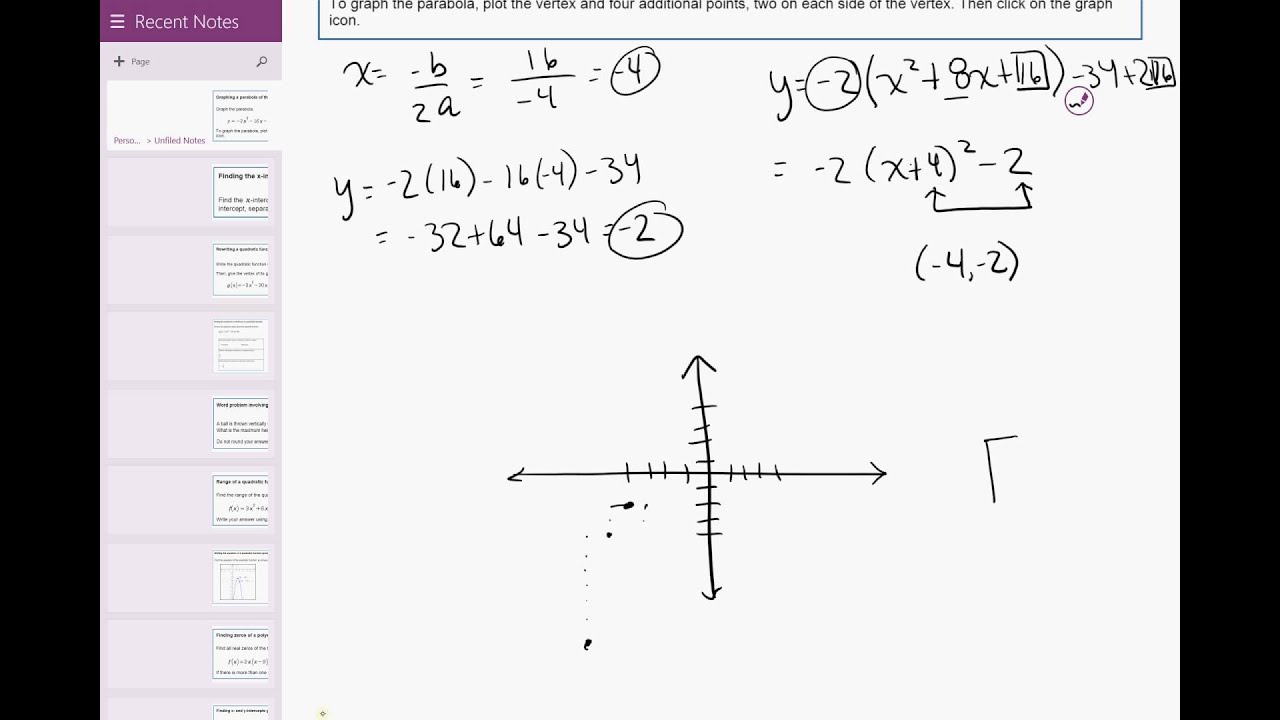



15 04 Graphing A Parabola Of The Form Y Ax 2 Bx C Integer Coefficients Youtube




View Question The Graph Of Y Ax 2 Bx C Is A Parabola With Vertical Axis Of Symmetry The Vertex Of This Parabola Is 2 3 And The Parabola Contain
Please Subscribe here, thank you!!!F (x) = ax 2 bx c are given by the quadratic formula The roots of a function are the xintercepts By definition, the ycoordinate of points lying on the xaxis is zero Therefore, to find the roots of a quadratic function, we set f (x) = 0, and solve the equation, ax 2 bx c = 0 We can do this by completing the square as,We want to find the vertex of this parabola The vertex is on the axis of symmetry, so its $x$coordinate is 3 The vertex is also a point on the parabola, so it satisfies the equation for the parabola This means that if you plug the $x$coordinate of the vertex into the equation, you will get the $y$coordinate Plugging 3 for $x$ into $y=x^26x$ gives $y=(3)^26(3)$ → $y=918$



Graphing Quadratic Equations Functions Parabolas By Finding Vertex Worksheet




Graphing Quadratic Functions Y Ax 2 Bx C Quadratic Functions The Graph Of A Quadratic Function Is A Parabola A Parabola Can Open Up Or Down If Pptx Powerpoint
Is it possible to generalize for any function f(x)? Focusing on the standard form of a parabola y = ax 2 bx c and the vertex equation y = a(x – h) 2 k, we can get the first formula of vertex ie The vertex formula will be (h, k) = (b/2a, D/4a) where D = b 2 – 4ac How do you Use Vertex Formula?PARABOLAS TRANSLATIONS AND APPLICATIONS QUADRATIC RELATION A quadratic relation in two variables is a relation that can be written in the form y=ax^2bxc or x=ay^2byc where a, b, and c are real numbers, and a!=0 The graphs of quadratic relations are called parabolas The simplest quadratic relation of the form y=ax^2bxc is y=x^2, with a=1, b=0, and c=0, so this
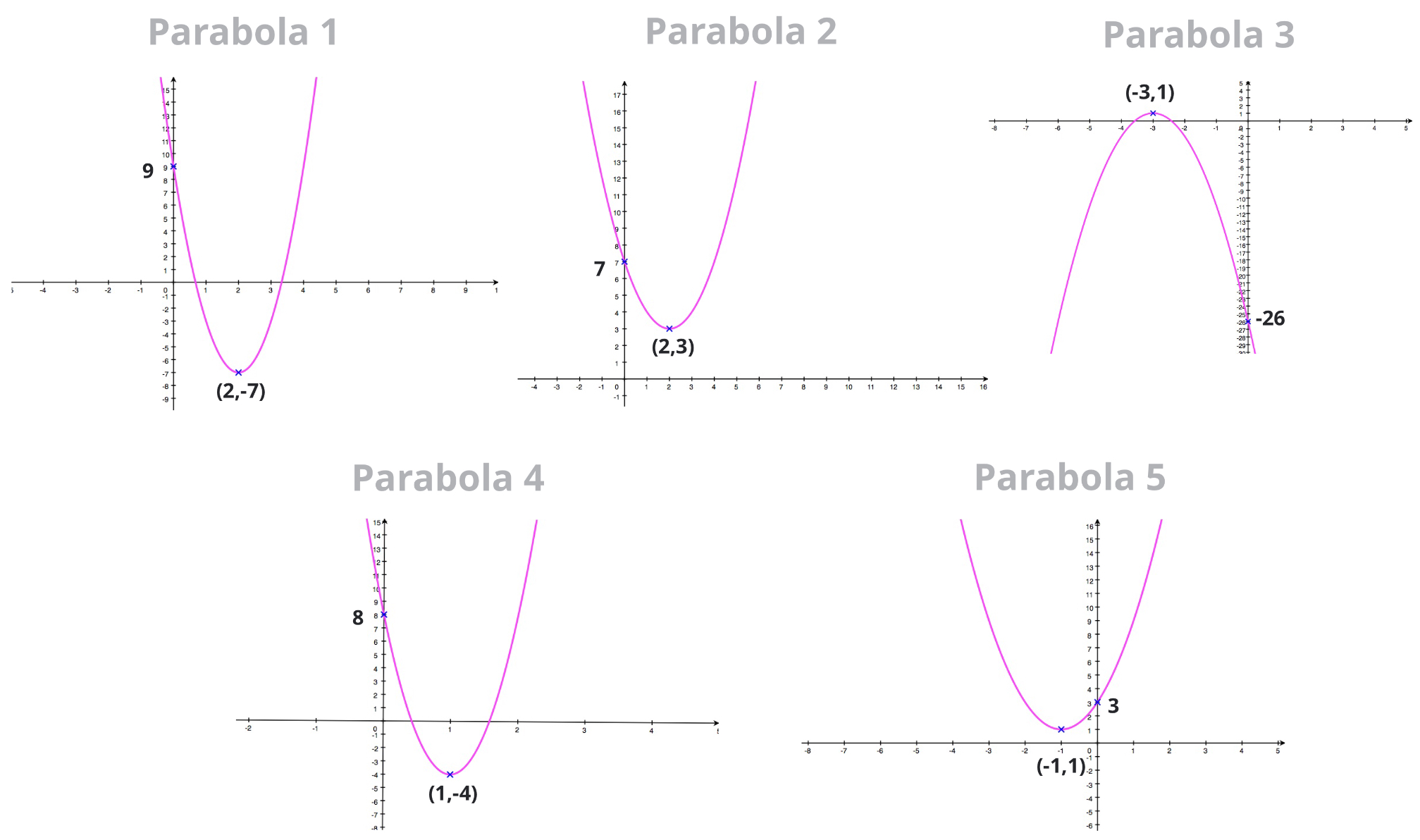



Vertex Form How To Find The Equation Of A Parabola




Quadratic Function Y Ax 2 Bx C Quadratic
y = ax 2 bx c The vertex of a parabola is the coordinate from which it takes the sharpest turn whereas a is the straightline used to generate the curve Focus is the point with is equidistant from all points of the parabola Here, we will find the vertex, focus, and directrix of a parabola There is a mathematical formula that finds allSOLUTION A parabola y = ax^2 bx c has vertex (4, 2) If (2, 0) is on the parabola, then find the value of abcThe general form of a quadratic is "y = ax 2 bx c"For graphing, the leading coefficient "a" indicates how "fat" or how "skinny" the parabola will beFor a > 1 (such as a = 3 or a = –4), the parabola will be "skinny", because it grows more quickly (three times as fast or four times as fast, respectively, in the case of our sample values




Graphing A Parabola Of The Form Y Ax2 Bx C With Integer Coefficients Youtube
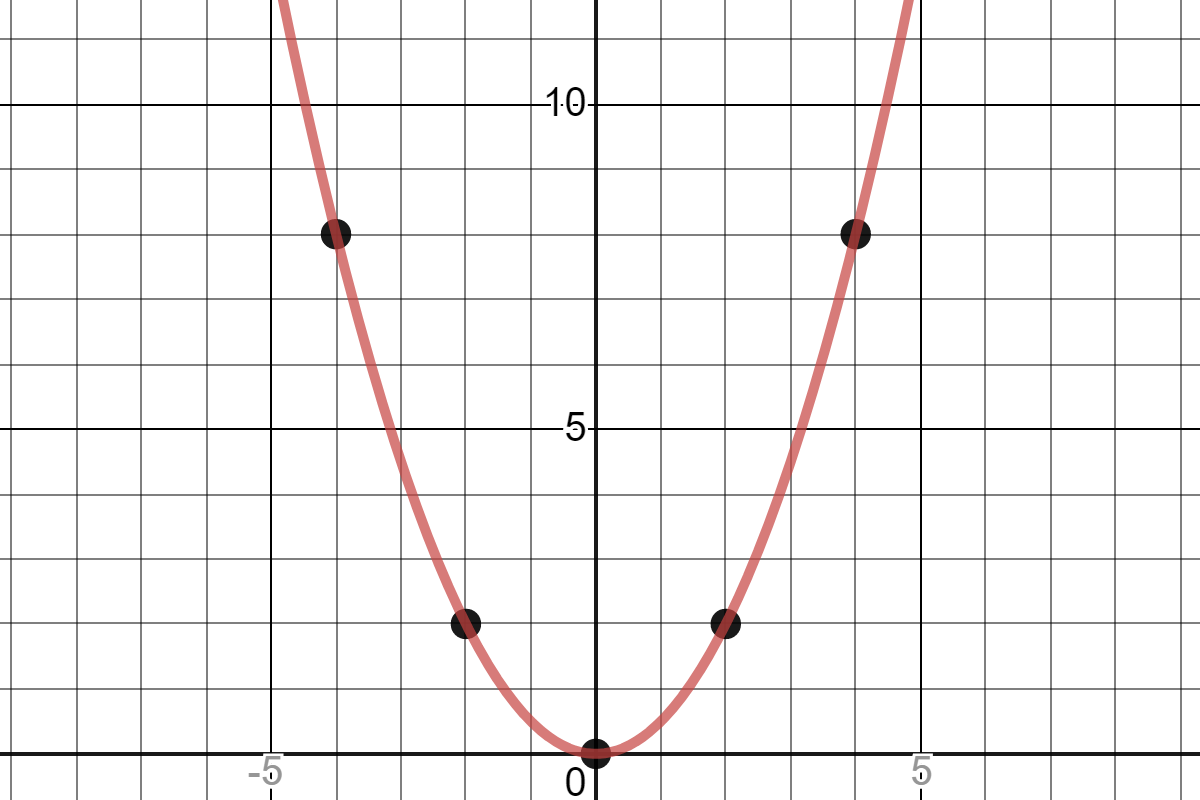



Quadratic Graph Example Y Ax Expii



0 件のコメント:
コメントを投稿